Concentration Effects And The Nernst Equation

The Nernst equation is the equation used to describe the reduction and oxidation that occur on the cell electrode, Nernst equation calculator guides us about the life of the cell. How to prolong the equilibrium persists. In electrochemistry, the equation relates the reduction potential of a reaction to the standard electrode potential. The equilibrium potential calculator describes the time of equilibrium that persists in the cell of the ox-red process.

The equation was discovered by German scientist Walter Nernst.

The concentration effect of the Nernst Equation can be explained by the following method:

The concentration of effect of the Nernst equation:

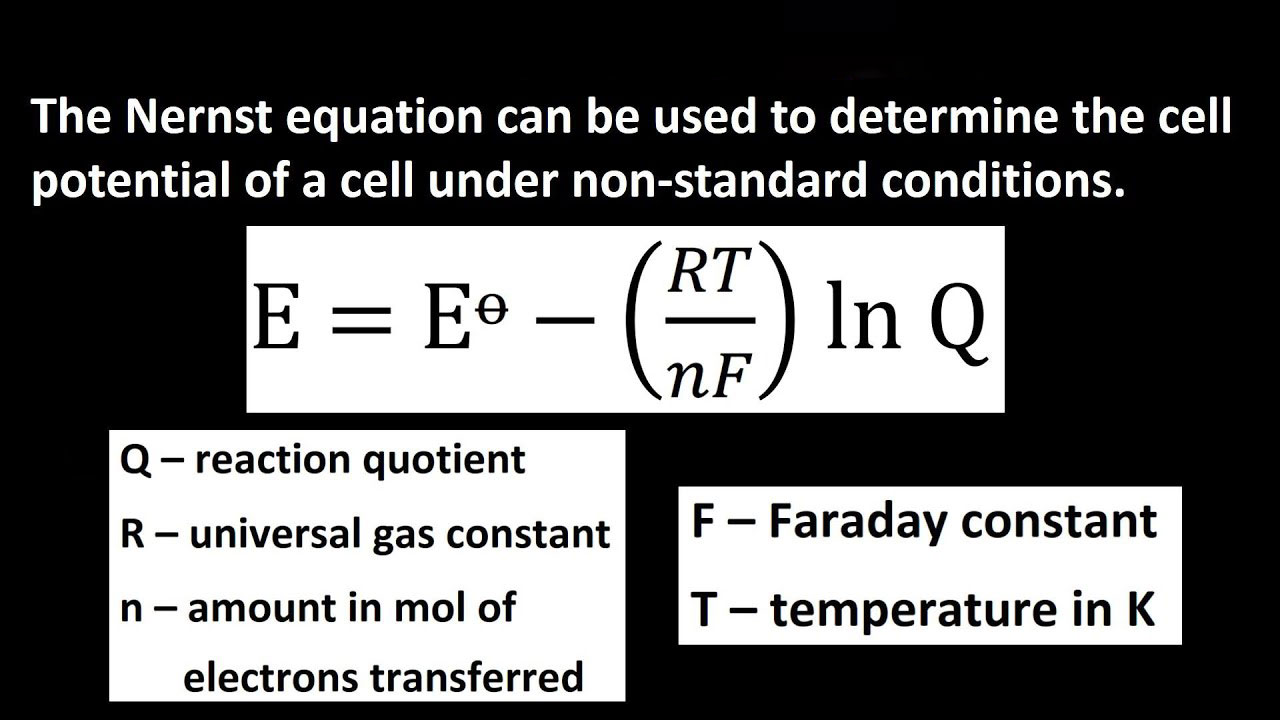
When we study the Nernst Equation it enables us to find the cell potential under non-standard conditions. 

You have to recall the actual free energy change for reaction under non-standard condition 

                                                ΔG=ΔG°+RTlnQ———— (1)

Where ΔG=−nFEcell, ΔG°= −nFE°cell under the non-standard condition:

Now substituting the values of the in the equation(1) we get:

                                        −nFEcell= −nFE°cell +RTlnQ

Now dividing both sides by −nF, we get:

                                           Ecell=E°cell- (RT/nF)lnQ———— (3)

Equation (3) is the Nernst equation. It is one of the most important in electrochemistry. You can use the  Nernst equation calculator, to find the values of the variables. You need to find out the values quickly during your calculation, to find the life of the cell.

  The uses of Nernst Equation:

There are different applications of the Nernst equation in analytical chemistry:

• The Nernst equation is used to find the nerve condition and to find the membrane potential of the human body.
• To find the life of electrochemical cells, and how to prolong would remain the equilibrium of the oxidation and the reduction process.
• Nernst equation is used to find the pH of chemicals, which is critical to determine the acidity and the alkalinity of the chemical.
• The Nernst equation is also used to find the solubility of the chemical.
• The find the values of the constant of equilibrium, the Nernst potential calculator can determine how to prolong the oxidation and reduction process, and how long the battery continues to work efficiently.
• It helps to find a relationship between the potential of the electrode and the potential of the standard electrode. 
• The Nernst equation predicts to which side the reaction will move in forward or in the reverse direction by determining the value of the equilibrium constant K.

Nernst equation and solubility:

We can find the solubility of the chemical by using the Nernst equation calculator. Nernst equation works efficiently where the low concentration of the ions is in equilibrium with sparingly soluble salt. The measurement of the concentration of the relevant ions can be calculated directly. It is more common to establish a cell, in which one electrode involves the accumulation of the insoluble salt during the reaction. It determines the solubility of the salt in water.

Example:

We can use the silver and silver chloride electrodes in a cell for the calculation of Ksp. The Ksp value predicts the molarity concentration of the silver ions of silver chloride.

The measurement of the pH:

The Nernst equation can be used to determine the pH of a chemical, The pH of a solution is the number of hydrogen ions in a solution. We can use a hydrogen electrode to find the direct activity of the hydrogen ions in a solution. The activity of the hydrogen ions can be expressed by an expression, the pH is used to determine how acidic and alkaline the solution is. The acidity of the solution is the highest when the value of the pH=1 and the solution is the alkaline if pH=14, the solution is neutral if solution pH=7 : 

                                            pH= -log aH+

Nernst equation is a useful equation to find various chemical properties.